Course Content
Study Resources
0/2
Quadratics
0/6
Completing the square for a quadratic polynomial
00:00
drawing and use quadratic graph
00:00
find the discriminant of a quadratic polynomial
00:00
solve quadratic equations, and quadratic inequalities, in one unknown
00:00
solve by substitution a pair of simultaneous equations of which one is linear and one is quadratic
00:00
recognise and solve equations in x which are quadratic in some function of x.
00:00
Functions
0/1
Coordinate geometry
Circular measure
Trigonometry
Series
Differentiation
Integration
Private: CAIE Alevel Pure Maths 1 (AS)
Content

Table of Contents

Syllabus

find the discriminant of a quadratic polynomial ax^2+ bx + c and use the discriminant

  • e.g. to determine the number of real roots of the equation ax^2 + bx + c = 0. Knowledge of the term ‘repeated root’ is included.

Determinant

  • The discriminant of a quadratic equation is a value calculated from the coefficients of the equation that provides information about the nature of the roots (or solutions) of the equation.
\Delta = b^2 - 4ac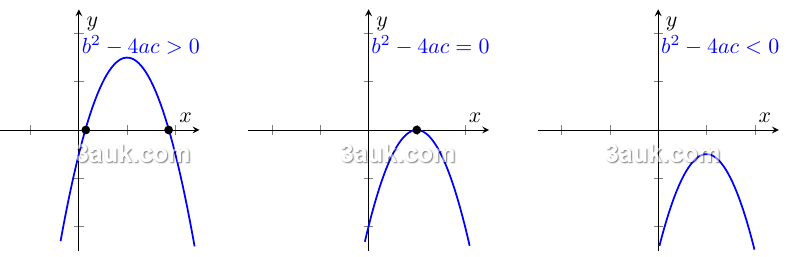

  • The value of the discriminant determines the number of real roots of the equation and their type. Specifically:
    • If the discriminant is positive, the equation has two real roots that are distinct and different.
    • If the discriminant is zero, the equation has exactly one real root that is a repeated root.
    • If the discriminant is negative, the equation has two complex roots that are conjugates of each other.
Spread the love
Join the conversation
Previous
Next